Video showing how to play MegaTangram
What are Tangram puzzles
Tangram is a game invented sometime in the Chinese Song dynasty (960–1127), although the Tan part of its name from the Tang dynasty of 618-906. The game was introduced to America in 1815 and later gained popularity in European countries during the 19th century. Tangram consists of geometric shapes called Tans (the gram part of the Tangram name refers to gramma the greek word for graph or picture).
Relative geometry of the tangram pieces
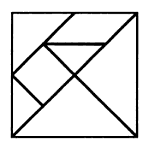
The tans are obtained from the division of a square into 7 distinct pieces, all shapes having either 45, 90 or 135 degree angles; 5 Isosceles Right Triangles a square and a parallelogram.
The medium sized triangle has its hypotenuse length the same as the shorter side of the larger triangle. The shorter side of the medium sized triangle is the same size as the smaller triangle hypotenuse and the longer side of the parallelogram. The length of the shorter size of the smaller triangle is half the length of the smaller size of the medium sized triangle and is equal to the shorter side of the parallelogram and the side of the square. The ratios between the sides in the tangram shapes are related to each other by sqrt(2). The ratio 1:sqrt(2) is used in A4 paper sizes and is chosen so that when the paper folded in half the aspect ratio is the same. The parallelogram is the only chiral piece (in the 2D plane), this means it cannot be transformed in to its mirror image form by rotations and translations alone and must be flipped over.
|
|
These geometrical properties of the Isosceles Right Triangles and other shapes that make up the Tangram puzzle mean that the shapes can be aligned adjacent to each other and produce a variety of interesting shapes and forms. As with all puzzles there are some unknowns that we must use brain power to deduce. In the case of the Tangram puzzle we are only given the shape outline and have to discover through logic or intuition the correct placement of the pieces to fit the shape. Tangram is a relatively easy puzzle to solve and hence is not particularly stressful to play and this, along with the many diverse and interesting designs, accounts for its long lasting popularity.
The MegaTangram online tangram game
MegaTangram is an online puzzle game with nearly 1950 puzzles to solve. By clicking on the button on the top right hand of the screen you can create your own tangram puzzle and also save it in your ejectamenta account (provided you are first logged on). The shapes can be moved by clicking and dragging the pieces across the board, if you click and drag on the edge of the piece the piece rotates. If you click on the edge of the piece they rotate by 45 degree except in the case of the parallelogram piece which flips over (see previous discussion on its chriality or handedness). The game has an “edge align” feature for the shape edges that helps with accurate alignment when the game is in this mode the cursor changes to crosshairs and shapes pause when they align with the edges of other shapes. To change between align and non-align mode click anywhere without moving, the cursor will change from the crosshair to a hand pointer and you can move the piece anywhere you want.
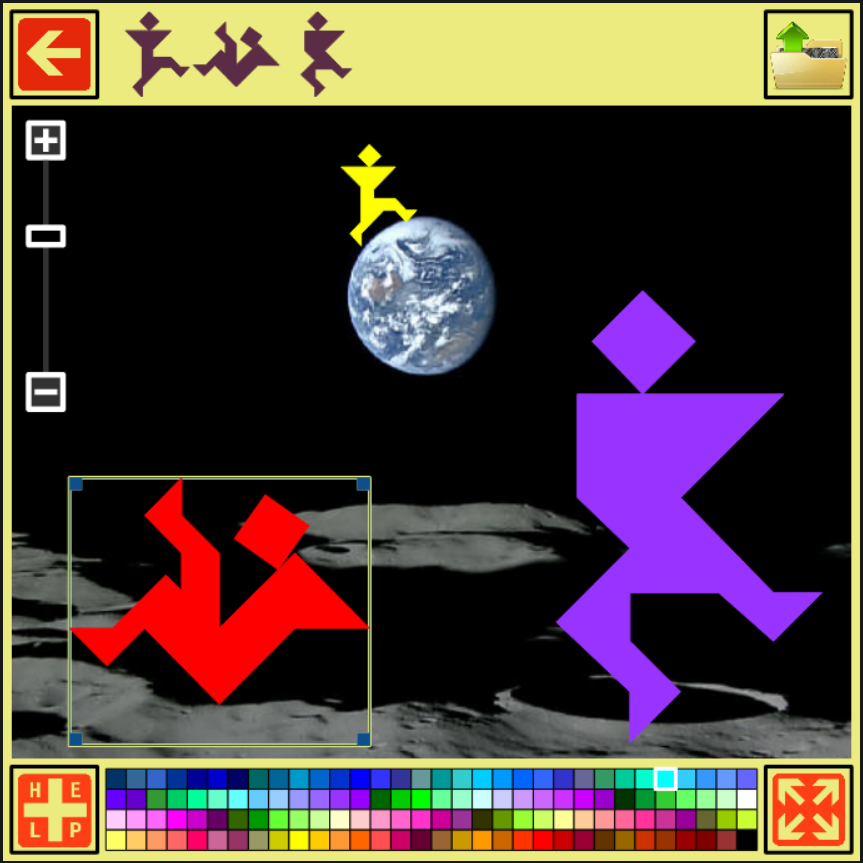
You can also zoom in or out, move the canvas or make the game fullscreen if you find that game play is a bit tight. Once a puzzle is solved it is saved in the user home page and also shows at the top of the tangram puzzle screen. If you click on any of these solved tangrams (or choose the Create Puzzle Landscape menu option) you are taken to a page in which you can add your completed tangrams to an image, colour them and build a landscape picture (an example is shown below).
Tangram Stanza
(Introduction from ‘the fashionable chinese puzzle’, Goodrich and Co. pub. 1818)
Farewell both to Chess and Backgammon! farewell too,
My earliest favourite, the Fox and the Geese!
At which, long before I could write, read, or spell too,
I’ve spent many hours of pastime and peace.
Now for squares and for rectangles, emblems of Science,
And for forms emblematical – none knows of what;
Like Fuseli’s dreams, bidding language defiance,
You can’t tell what they are – no, nor what they are not.
But nor Poet, nor Painter, though giving free scope to
The visions of fancy, when fancy is warm,
Let them do all they can, yet they never could hope to
Surpass the queer figures your Puzzles can form.
Each Beauty, with finger so fair and so taper,
Replaces each section again and again;
Then poutingly turns from the toy to the paper,
Half angry to find that her toil is in vain.
The Geometrician sagaciously chooses
By rule and by reason each piece he lays down;
But still now and then his sage labour he loses,
While he strives to look wiser by dint of a frown.
But He* most of all, holds in due estimation
Your puzzle so peerless, who, harass’d with care,
Has recourse to its aid as a mere recreation,
Which attracts all his thoughts, and which drives off despair.
There are moments in which reading is no relaxation;
When music’s enchantments wake only a sigh;
When all the bright beauties which bloom in creation,
Flash in vain on the fancy, and charm not the eye:
There are hours, in short, when one’s flagg’d spirits sinking
In that kind of employment alone can find ease,
Which so rivets our thoughts we scarce know we are thinking,
And in such moody moments your puzzle can please.
* This ingenious contrivance has for some time past been the favourite amusement of the Ex-Emperor Napolean, who, being now in a debilitated state, and living very retired, passes many hours a day in thus exercising his patience and ingenuity.